Numerical Method
One shot Playlist- NSG
Estimated Marks : 40
This is One shot videos playlist by Ashish Panta (Nepali Science Guru), however this does not cover PDE. If you are just watching before exam, quickly checkout these videos and practice questions. If you have time remaining then you can dedicately checkout chapterwise videos.
 Error and Non-linear Equations
Error and Non-linear Equations Interpolation
Interpolation Differentiation & Integration
Differentiation & Integration System of Linear Eqns
System of Linear Eqns Ordinary Differential Eqns
Ordinary Differential Eqns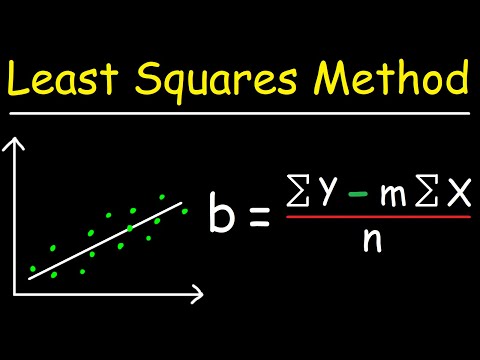 Linear Regression
Linear Regression- 1Error and Non-linear Equations
- 2Interpolation
- 3Differentiation & Integration
- 4System of Linear Eqns
- 5Ordinary Differential Eqns
- 6Linear Regression
Error
Estimated Marks : 5
This is fundamental chapter which means that this builds a lot of base for next chapters. Relatively this chapter is very easy. You must know terms like True Errors, Relative Errors, Approximate Errors before you go to next chapters. Also basic of taylor series will help in dervations as well.
 Numerical Method - Intro
Numerical Method - Intro Error
Error- 1Numerical Method - Intro
- 2Error
Non-linear Equations
Estimated Marks : 10
10 marks from this chapter usually (surely 5 marks atleast). In Numerical method, you will learn different methods to solve different problems. For each problem, you must learn method and algorithm (program also for practical). In this chapter you will learn how to solve non-linear equations with algorithm (keep that in mind).
 Intro
Intro Bisection Method
Bisection Method Newton Raphson Method
Newton Raphson Method Secant Method
Secant Method Fixed Point Method
Fixed Point Method Horners Method
Horners Method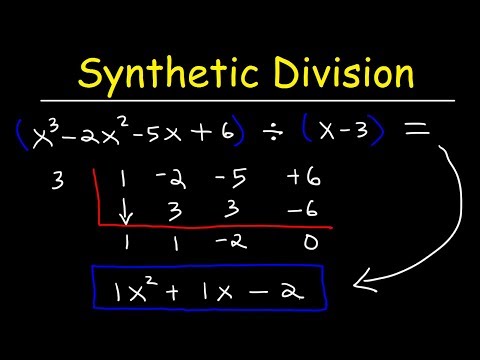 Synthetic Division
Synthetic Division- 1Intro
- 2Bisection Method
- 3Newton Raphson Method
- 4Secant Method
- 5Fixed Point Method
- 6Horners Method
- 7Synthetic Division
Interpolation
Estimated Marks : 15
Interpolation means fitting a curve exactly through given points. This chapter is fairly difficult. Netwon Divided Difference method is asked frequently in exam. Spline Interpolation is a bit more harder.
 Interpolation - One shot
Interpolation - One shot- 1Interpolation - One shot
Regression
Estimated Marks : 5
Very easy compared to other chapters. Sure 5 marks in exam after you learn 2 main topics: Linear Regression (mainly this) and Non linear Regression. You might know Linear regression from class 12.
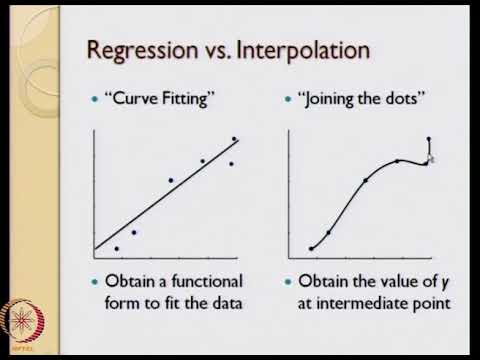 Interpolation vs Regression
Interpolation vs Regression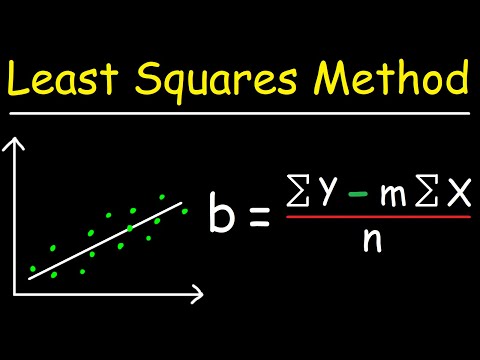 Linear Regression
Linear Regression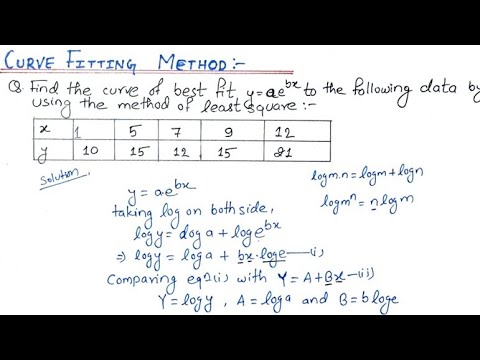 Exponential Regression
Exponential Regression Polynomial Regression
Polynomial Regression- 1Interpolation vs Regression
- 2Linear Regression
- 3Exponential Regression
- 4Polynomial Regression
System of Linear Equations
Estimated Marks : 15
Sure 15 marks (rarely 20 marks). Many topics but they are fairly easy. You must spend sometime and practice some questions. Be sure to grab 15 marks from this chapter in exam.
 Consistent vs Inconsistent
Consistent vs Inconsistent Gauss Elimination
Gauss Elimination Gauss Jordan Method
Gauss Jordan Method Matrix Inversion
Matrix Inversion LU Factorization
LU Factorization Cholesky Method
Cholesky Method Jacobi Method
Jacobi Method Gauss Siedel Method
Gauss Siedel Method Ill Conditioning
Ill Conditioning Eigen V. - Power Method
Eigen V. - Power Method  Eigen V. - Inverse Power Method
Eigen V. - Inverse Power Method- 1Consistent vs Inconsistent
- 2Gauss Elimination
- 3Gauss Jordan Method
- 4Matrix Inversion
- 5LU Factorization
- 6Cholesky Method
- 7Jacobi Method
- 8Gauss Siedel Method
- 9Ill Conditioning
- 10Eigen V. - Power Method
- 11Eigen V. - Inverse Power Method
Differentiation
Estimated Marks : 5
Newton Divided Difference method from chapter 3 is necessary to understand this chapter. This chapter is small and easy, but not much asked in exam. Content is not much available for it on youtube. 2nd video will give you basic understanding then you can checkout out remaining videos where particular method is explained with examples.
 Quick - Basics
Quick - Basics For Unequal Interval
For Unequal Interval Tabulated Data
Tabulated Data Differential - More examples
Differential - More examples Minima & Maxima
Minima & Maxima- 1Quick - Basics
- 2For Unequal Interval
- 3Tabulated Data
- 4Differential - More examples
- 5Minima & Maxima
Integration
Estimated Marks : 10
This is a important chapter for exam. But some guidelines will help you here. Basically, there are three types of integration methods: Newton Cote's Formula, Gaussian Formula & Romberg Formula. Newton Cote's is parent formula for Trapezoidal & Simpsons 1/3 and 3/8 rule. For exam the derivation is not required, learn the Trapezoidal and Simpson's formula. Gaussian and Romberg dont have derived formulas like Newton Cotes. We use Newton Cote's Derived formula when the interval is equally spaced else we use Gaussian or Romberg. Also while watching video of Gaussian or Romberg, have some patience since it is new concept, just learn how to use them in exam.
 Newton Cote's Formula & Concept
Newton Cote's Formula & Concept Trapezoidal, Simpsons 1/3 & 3/8
Trapezoidal, Simpsons 1/3 & 3/8 Gauss Integration - Concept & Process
Gauss Integration - Concept & Process Gauss Integration - Practice
Gauss Integration - Practice Romberg Integration
Romberg Integration- 1Newton Cote's Formula & Concept
- 2Trapezoidal, Simpsons 1/3 & 3/8
- 3Gauss Integration - Concept & Process
- 4Gauss Integration - Practice
- 5Romberg Integration
Ordinary Differential Equations
Estimated Marks : 10
A slight confusion that might occur is that Heuns Method is also called Modified Eulers Method. Also System of ODE and higher order equations can be solved by different methods like Euler, Picard, Taylor, just because the video uploaded shows by one method does not mean they can't be solved using other. Practice a lot. Check past year questions also (maybe available soon).
 Taylor Series Method
Taylor Series Method Taylor Series - Practice
Taylor Series - Practice Picard Method
Picard Method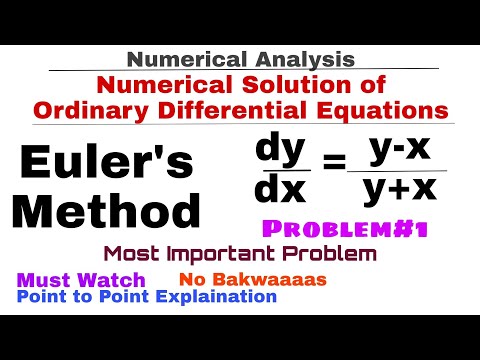 Eulers Method
Eulers Method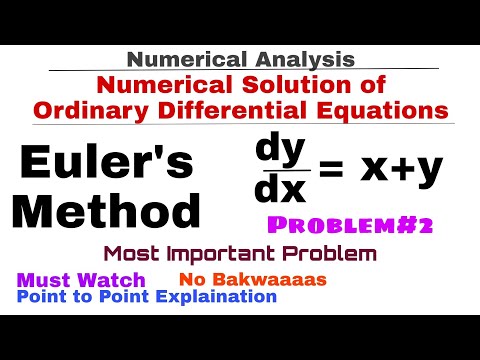 Eulers Method - Practice
Eulers Method - Practice Heuns Method
Heuns Method Runge-Kutta 2nd Order
Runge-Kutta 2nd Order Runge-Kutta 4th Order
Runge-Kutta 4th Order System of ODE
System of ODE Higher Order Differential
Higher Order Differential Shooting Method
Shooting Method- 1Taylor Series Method
- 2Taylor Series - Practice
- 3Picard Method
- 4Eulers Method
- 5Eulers Method - Practice
- 6Heuns Method
- 7Runge-Kutta 2nd Order
- 8Runge-Kutta 4th Order
- 9System of ODE
- 10Higher Order Differential
- 11Shooting Method
Partial Differential Equations
Estimated Marks : 5
Good content for this chapter is hard for us to find. Also besides Possion Equation Problem, frequent problem is not seen in exam. (If you have found videos that suits the course, provide the link through feedback)